Search Sanlev

Computational Applications

Home/Aluminium Academy/Computational Applications/Triangle Solution 1

Triangle Solution 1

Using our computing applications you can easily find the weight per meter of a cross section of aluminum, steel or any other material, entering its density in the appropriate cell.

A very useful feature available to you is to find the weight of your order by entering the number of items and their length.

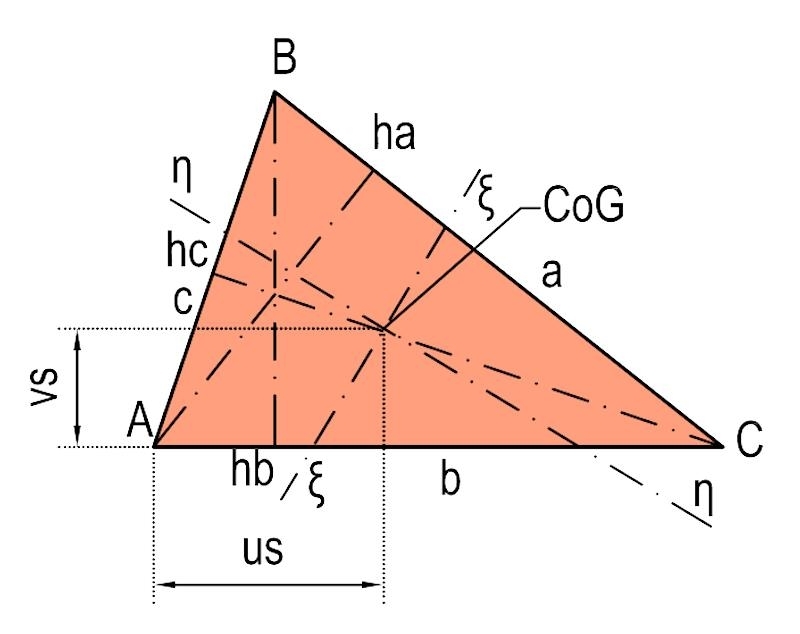
Also, you can easily find the surface of the cross section, the center of gravity, the moments of inertia, the moments of resistance and many other properties of the cross section used in structural calculations and material strength.
Data
Density of Alloy (kgr/m3):
Aluminium
Steel
Other
Side a:
Side b:
Side c:
Profile Length (mm):
Number of Pieces (pcs):
Results
Weight (gr/m):
Side a:
Side b:
Side c:
Angle a:
Angle b:
Angle b:
Height of side a:
Height of side b:
Height of side c:
Interstitial side a:
Interstitial side b:
Interstitial side c:
Angle ö:
Triangle Area:
Perimeter (mm):
È Helper::
Radius of inscribed circle:
Radius of circumcircle:
Moment of Inertia Jx ê.â. (cm4):
Moment of Inertia Jy ê.â. (cm4):
Moment of Inertia Jxy (cm4):
Radius Inertia ix (cm3):
Weight (kg):
Radius Inertia iy (cm3):
Radius Inertia ixy (cm3):
X centroid (mm):
Õ centroid (mm):
Moment of Inertia Jx (cm4):
Moment of Inertia Jy (cm4):
Moment of Inertia Jxy (cm4):
Principal moments of inertia K.B:
J11 (cm4):
J22 (cm4):
È(deg) rotation angle from largest moment of inertia:
Cookie Preferences
Consent
Details
About
This website uses cookies
We use cookies to personalise content and ads, to provide social media features and to analyse our traffic. We also share information about your use of our site with our social media, advertising and analytics partners who may combine it with other information that you¢ve provided to them or that they¢ve collected from your use of their services.
Customize preferences